Question

The measure of an angle is nine times the measure of its complement. find the measure of each angle.

Answer 1

The measure of the angle is 81°, and the measure of its complement is 9°.

Explanation:

Problem Statement:

The measure of an angle is nine times the measure of its complement. Find the measure of each angle.

Let's call the measure of the angle "θ" degrees and the measure of its complement "θ_c" degrees.

`\hrulefill`

According to the problem statement, we're given that the measure of the angle θ is nine times the measure of its complement θ_c. We can write this relationship as an equation:

`\Longrightarrow \theta_c = (\theta)/(9)`

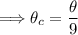
Since the sum of an angle and its complement is 90 degrees, we can write another equation:

`\Longrightarrow \theta + \theta_c=90\textdegree`

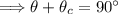
Now we have a system of two equations with two unknowns (θ and θ_c):

`\Longrightarrow \left\{\begin{array}{ccc}\theta_c=(\theta)/(9) \\\theta+\theta_c=90\textdegree\end{array}\right`

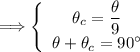
Substitute the value of θ_c from the first equation into the second equation:

`\Longrightarrow \theta+ (\theta)/(9) =90 \textdegree`

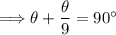
Combine like terms:

`\Longrightarrow (10)/(9) \theta =90 \textdegree`

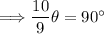
Multiply both sides by 9/10:

`\Longrightarrow \theta =90 \textdegree \cdot (9)/(10) \\\\\\\\\therefore \boxed{\boxed{\theta = 81 \textdegree}}`

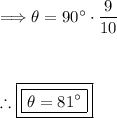
Now that we know the value of the angle is 81 degrees, we can find the value of the complement θ_c using the first equation:

`\Longrightarrow \theta_c= (81\textdegree)/(9) \\\\\\\\\therefore \boxed{\boxed{\theta_c=9 \textdegree}}`

Thus, the measure of the angle is 81 degrees, and the measure of its complement is 9 degrees.

`\hrulefill`

Additional Information:

System of Equations: This problem involves solving a system of equations, which is a set of equations with multiple variables. The goal is to find the values of the variables that satisfy all the equations simultaneously.

Complementary Angles: Complementary angles are pairs of angles that add up to 90 degrees when combined. In other words, if you have two angles, and their measures, when added together, result in 90 degrees, those angles are said to be complementary to each other.

Answer 2

Answer: 9° and 81°

Explanation:

We know that complement angles are equal to 90 degrees when added together. Using this information, along with the fact that one angle is nine times the other, we can create an equation to solve for the measure of the angles. Let x be the measure of one of the angles.

Given:

90° - x = 9x

Add x to both sides of the equation:

90° = 10x

Divide both sides of the equation by 10:

9° = x

Lastly, we will substitute this into the expression representing the other angle to solve, knowing now that one of the angles is equal to 9°.

Given:

9x

Substitute into the expression:

9(9°)

Multiply:

81°