Question

A rectangle's length is 4 feet more than its width. write a quadratic function that expresses the rectangle's area in terms of its width.

Answer 1

Let's call the width: w and the length: L.

L = w + 4
Area = L x w

Substitute w+4 for L in the area equation.

So, Area = (w+4) x w
Rewritten as: w^2 + 4w

Answer 2

Answer: The answer is
`A=w^2+4w.`

Step-by-step explanation: Given that the length of a rectangle is 4 feet greater than its width. We are to write a quadratic function that expresses the area of the rectangle in terms of its width.

Let 'w' represents the width of the rectangle. Then the length of the rectangle will be (w + 4).

Therefore, The area of the rectangle will be

`A=w* (w+4)\\\\\Rightarrow A=w^2+4w.`

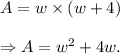
Thus, the answer is
`A=w^2+4w.`