Question

How do you complete the square using fractions

Answer 1

alrighty
we will complete the squaer for ax²+bx+c=0 regardless of the values of a,b, or c
we will factor it into a(x-h)²=r

so
first group x terms
(ax²+bx)+c=0
factor out a

`a(x^2+(b)/(a)x)+c=0`
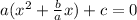
take 1/2 of the linear coefinet and squaer it

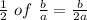
`(1)/(2) \space\ of \space\ (b)/(a)=(b)/(2a)` square it to get
`(b^2)/(4a^2)`
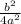
add positive and negative of that inside parntheasees

`a(x^2+(b)/(a)x+(b^2)/(4a^2)-(b^2)/(4a^2))+c=0`
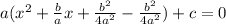
factor perfect square

`a((x+(b)/(2a))^2-(b^2)/(4a^2))+c=0`
expand

`a(x+(b)/(2a))^2-(b^2)/(4a)+c=0`
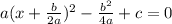
and that's how you complete the square, just move the constants over to the left when you're done then divide both sides by a then square root both sides, remembering to take the positive and negative roots