Question

A student has some 1 and 5 bills in his wallet. he has a total of 14 bills that are worth 50 how many of each type of bill does he hae

Answer 1

There were 5 bills of '1' and 9 bills of '5'.

Explanation:

let the number of '1' bill be x

let the number of '5' bill be y.

Total number of bills = 14

x + y = 14 ..[1]

Worth of 14 bills = 50

So,
`x* 1+y* 5=\$50`

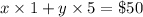
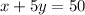`x+5y=50`..[2]

x + y = 14

x = 14 - y

Putting value of x in [2]:

`14- y +5y=50`

`y=(50-14)/(4)=9`

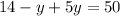
y = 9

x = 14-y = 14 - 9 = 5

There were 5 bills of '1' and 9 bills of '5'.

Answer 2

x = ones

y = fives

x+y = 14 total bills

rewrite as x = 14-y

1x +5y = 50

1(14-y) +5y = 50

14-1y +5y = 50

14 +4y = 50

4y = 36

y = 36/4 = 9

x = 14-y = 14-9 = 5

9+5 = 14

9x5 = 45 +5x1 = 50

he had 9 fives and 5 ones