Question

What is the maximum height of the ball ?har far from the child does the ball strike the ground ?

Answer 1

a. y = 3 feet

b. The maximum height is 19 feet

c. 33.44 ft

EXPLANATION :

From the problem, we have the equation :

`y=-(1)/(16)x^2+2x+3`

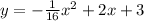
a. The height of the ball, y when x = 0 is :

`\begin{gathered} y=-(1)/(16)(0)^2+2(0)+3 \\ \\ y=3 \end{gathered}`

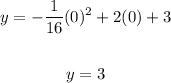
b. The maximum height can be obtained by substitute x = h

where h is :

`h=-(b)/(2a)`

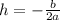
Solve for h with a = -1/16 and b = 2

`\begin{gathered} h=-(2)/(2(-(1)/(16))) \\ \\ h=16 \end{gathered}`

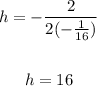
Substitute x = h = 16, then solve for y :

`\begin{gathered} y=-(1)/(16)(16)^2+2(16)+3 \\ \\ y=19 \end{gathered}`

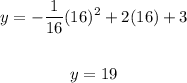
c. The distance of the ball to the child when the ball strikes the ground is when y = 0

`\begin{gathered} y=-(1)/(16)x^2+2x+3 \\ \\ 0=-(1)/(16)x^2+2x+3 \end{gathered}`

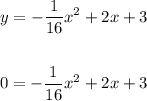
Using quadratic formula with a = -1/16, b = 2 and c = 3

`\begin{gathered} x=(-b\pm√(b^2-4ac))/(2a) \\ \\ x=\frac{-2\pm\sqrt{2^2-4(-(1)/(16))(3)}}{2(-(1)/(16))} \\ \\ x=\frac{-2\pm\sqrt{(19)/(4)}}{-(1)/(8)} \\ \\ x=(-2\pm(1)/(2)√(19))/(-(1)/(8)) \\ \\ x=16\pm4√(19) \\ \\ \text{ In decimals,} \\ \\ x=16+4√(19)=33.44\text{ }ft \\ x=16-4√(19)=-1.44\text{ }ft \end{gathered}`

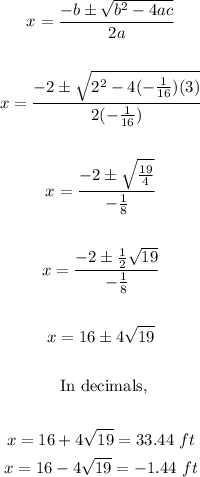
Since there's no negative distance, the answer will be 33.44 ft