Question

7 The daily cost of hiring a plumber, y, to work x hours on a repair project can be modeled using a linear function. The plumber charges a fixed cost of $80 plus an additional cost of $45 per hour. The plumber works a maximum of 8 hours per day. For one day of work, what is the range of the function for this situation? A 0

Answer 1

Choice B. 80 < y < 440

Step-by-step explanation:

The information given can be summarized in the following equation

`y=80+45x`

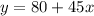
where y is the daily cost and x is the number of hours worked.

The range of aa function is the set of all possible values it can take. In our context, the range is the possible "range" of a plumbers earnings.

Now, the minimum a plumber can earn daily is by working 0 hours. Then the worker charges

`\begin{gathered} y=80+45(0) \\ y=80 \end{gathered}`

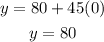
The plumber charges $80.

Moreover, the maximum a worker can charge is when they work 8 hours (which is the maximum number of hours they can work)

The cost of working 8 hours is

`\begin{gathered} y=80+45(8) \\ y=440 \end{gathered}`

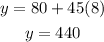
This means that the plumber can earn between $80 and $440 per day and this statement can be written as

`80\le y\le440`

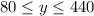
Hence, the range of the function is given by choice B.