Question

Find the product of the complex number and its conjugate. 1 + 2i

Answer 1

The answer to this question is:


Find the product of the complex number and its conjugate. 1 + 2i ??(1+2i)
(1-2i)=
1^2+2^2=
5

Hoped This Helped, Trevorhardy
Your Welcome :)

Answer 2

5

Explanation:

If a complex number is defined as a+bi, then its conjugate is defined as

`\overline{z}=a-bi`

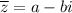
and the product of the complex number and its conjugate is

`z\overline{z}=(a+bi)(a-bi)=a^2+b^2`

The given complex number is

`z=1+2i`

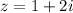
The conjugate of given number is

`\overline{z}=1-2i`

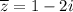
The product of the complex number and its conjugate is

`(1+2i)(1-2i)=1^2+2^2=1+4=5`

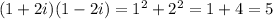
Therefore, the product of the complex number and its conjugate is 5.