Answer:
√65 units.
Step-by-step explanation:
The distance between two points (x1,y1) and (x2,y2) on the coordinate plane is calculated using the formula below:
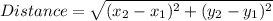
Given the points: (-4,-1) and (3,3).
![\begin{gathered} (x_1,y_1)=(-4,-1) \\ (x_2,y_2)=(3,3) \\ \implies Distance=\sqrt[]{(3-(-4))^2+(3-(-1))^2} \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/mbq2oy14ekci1c9g5rcw.png)
We simplify:
![\begin{gathered} Distance=\sqrt[]{(3+4)^2+(3+1)^2}=\sqrt[]{7^2+4^2}=\sqrt[]{49+16} \\ =\sqrt[]{65} \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/mat58oixolq81weq5sku.png)
The distance between the two points is √65 units.