Question

What function best represents a sine function with an amplitude of 4, a period of pi/6 , and a midline at y = −2?

A. f(x) = −2 sin(x − π/6 ) + 4


B. f(x) = 4 sin 12x − 2


C. f(x) = 4 sin(x − π/6 ) − 2


D. f(x) = −2 sin 12x + 4

Answer 1

The answer is B

f(x) = 4 Sin 12x - 2

Answer 2

B). f(x) = 4 sin 12x − 2.

Explanation:

Given : A sine function with an amplitude of 4, a period of pi/6 , and a midline at y = −2.

To find : What function best represents a sine function.

Solution : We have amplitude of 4, a period of pi/6 , and a midline at y = −2.

Standard form of sin function : y = a sin[ b(x-h)] + k.

Where, a = amplitude

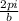`(2 pi)/(b)` = time period.

b =
`(2 pi)/(pi/6)`

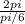
b = 12x

k = vertical displacement. , h = 0

Then Substitute the values a =4 , b = 12x k = -2 in standard form

y = 4 sin 12x - 2.

Therefore, B). f(x) = 4 sin 12x − 2.