Question

URGENT URGENT HELP In what direction and by how many units is the graph of f(x) = 6 sin(2x + π) − 5 vertically and horizontally shifted?

Answer 1

A. Down 5, Left pi/2

Answer 2

The required result is 5 unit vertically shifted downward and
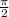`(\pi)/(2)` unit horizontally shifted left.

Explanation:

Given : Graph
`f(x) = 6\sin(2x+\pi)-5`

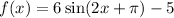
To find : In what direction and by how many units is the graph vertically and horizontally shifted?

Solution :

Vertically shift is up or down,

Vertically shifting down is shifting outside the function,

i.e, f(x)→f(x)-b

In the given graph, The graph is 5 unit vertically shifted downward as

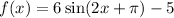`f(x) = 6\sin(2x+\pi)-5` i.e, 5 unit shifted downward.

Horizontal shift is either left or right,

Horizontally shift left is shifting inside the function,

i.e, f(x)→f(x+b)

We can write the given function as
`f(x) = 6\sin(x+(\pi)/(2))-5`

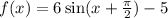
In the given graph, The graph is
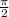`(\pi)/(2)` unit horizontally shifted left as

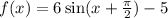`f(x) = 6\sin(x+(\pi)/(2))-5` i.e,
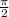`(\pi)/(2)` unit shifted left.

Therefore, The required result is 5 unit vertically shifted downward and
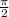`(\pi)/(2)` unit horizontally shifted left.