Question

Pensils are sold in packages of 10 and erasers are sold in packages of 6. What is the least number of pensils and erasers you can buy so that there is one pencil for each eraser with none left over.

Answer 1

To understand the problem consider the following cases.

i)

If we buy 1 packet of pencils, and 2 packet of erasers,

we have 1*10=10 pencils and 2*6=12 erasers.

ii)

If we buy 3 packet of pencils, and 4 packet of erasers,

we have 3*10=30 pencils and 4*6=24 erasers.


So let a and b be the correct number of packets of pencils and erasers respectively.

That is the least numbers a and b, such that 10a=6b.

10a=6b

divide both sides by 10:


`a= (6b)/(10)= (3b)/(5)`

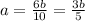
divide both sides by b:


`(a)/(b) = (3)/(5)`

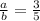
the ratio a:b cannot be simplified any further. This means that the smallest (natural) numbers a and b such that
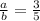
`(a)/(b) = (3)/(5)` are a=3 and b=5


Answer:


3 packages of pencils, 5 packages of erasers.