Question

A ball is thrown from a height of 40 meters with an initial downward velocity of 10/ms . The ball's height h (in meters) after t seconds is given by the following. =h−40−10t5t2 How long after the ball is thrown does it hit the ground?

Answer 1

h=-5t^2+10t+40
-5t^2+10t+40=0
t^2-2t-8=0
(t-4)(t+2)=0
t=4 s

Answer 2

After 2 seconds the ball will hit the ground.

Explanation:

A ball is thrown from a height of 40 meters with an initial downward velocity of 10/ms .

The ball's height h (in meters) after t seconds is given by the function

`h=40-10t-5t^2`

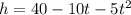
At ground level height of ball is 0.

`0=40-10t-5t^2`

`0=5(8-2t-t^2)`

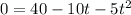
Splitting the middle term.

`0=5(8-4t+2t-t^2)`

`0=5(4(2-t)+t(2-t))`

`0=5(2-t)(4+t)`

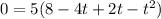
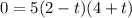
Using zero product property we get

`2-t=0\Rightarrow t=2`

`4+t=0\Rightarrow t=-4`

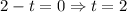
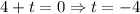
The time can not be negative. So, the value of t is 2

Therefore after 2 seconds the ball will hit the ground.