Answer:
C) The student did not properly simplify all radicals.
Explanation:
We are given the following expression in the question:

We have to apply the distributive property.
Distributive Property:
- The distributive property helps multiply a sum by multiplying each addend separately and then add the products.
- (a+b)(c+d) = ac + ad + bc + bd
Applying the distributive property:
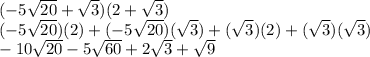
This can be further simplified as:
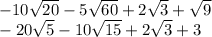
The student simplified the radical as:
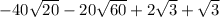
The student did a mistake in simplifying the radical. He did not solve the square roots properly.
C) The student did not properly simplify all radicals.