Question

The table of values below shows the rate of water consumption in gallons per hour at selected time intervals from t = 0 to t = 12.

Using a left Riemann sum with 5 subintervals, estimate the total amount of water consumed in that time interval.

x 0 2 5 7 11 12
f(x) 5.7 5.0 2.0 1.2 0.6 0.4

Answer 1

The answer is 35.8

Explanation:

You are using left riemann sum, meaning you take the area of each rectangle by the left side. Multiply the widths by heights then add together:

2(5.7) + 3(5.0) + 2(2.0) + 4(1.2) + 1(0.6)

=35.8

Answer 2

The total amount of water consumed in that time interval is 35.8.

Explanation:

A Riemann sum is used to find the approximate area under a curve by dividing it into multiple rectangular shapes.

The height of each rectangle in these multiple shapes is equal to the value of the function at the right endpoint of the base of rectangle.

The formula of Riemann sum is

`A=\int\limits^b_a {f(x)\Delta x_i} \, dx`

`A=f(0)(2-0)+f(2)(5-2)+f(5)(7-5)+f(7)(11-7)+f(11)(12-11)`

`A=(5.7)(2)+(5.0)(3)+(2.0)(2)+(1.2)(4)+(0.6)(1)`

`A=11.4+15+4+4.8+0.6`

`A=35.8`

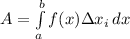
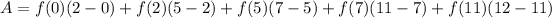
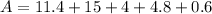
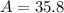
Therefore the total amount of water consumed in that time interval is 35.8.