Question

Find the sum of this infinite geometric series where a1=0.3 and r=0.55

Answer 1

Hello, Marymack!


We know that in a geometric sequence defined by
`\mathsf{a_1}` and
`\mathsf{r}` , the sum can be calculated the following way:


`\mathsf{S_n = (a_1)/(1-r)}\\ \\ \\ \\ \mathsf{S_n = (0.3)/(1-0.55)}\\ \\ \\ \mathsf{S_n = (0.3)/(0.45)}\\ \\ \\ \boxed{\mathsf{S_n = (2)/(3)=0.666...}}`

Answer 2

The sum of the infinite geometric sequence is given by:

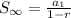`S_(\infty) = (a_1)/(1-r)` ....[1]

where,

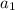`a_1` is the first term

r is the common ratio.

As per the statement:

Given that
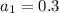`a_1 = 0.3` and r = 0.55

To find the sum of this infinite geometric series.

Substitute the given values in [1] we have;

`S_(\infty) = (0.3)/(1-0.55)`

⇒
`S_(\infty) = (0.3)/(0.45)`

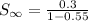
Simplify:

`S_(\infty) \approx 0.67`

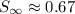
Therefore, the sum of this infinite geometric series approximate is, 0.67