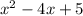Question

Select the correct answer from the drop-down menu.The values I= 2 + i are the roots of the quadratic equation

Answer 1

Given the question in the image, the following are the solution steps to answer the question.

STEP 1: Write the given roots

`x=2\pm i`

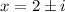
This means that the roots are:

`\begin{gathered} x=2+i \\ x=2-i \end{gathered}`

STEP 2: Write the form of getting a quadratic equation using the roots

`\begin{gathered} if\text{ }x=a,b\text{ is a root then,} \\ (x-a)(x-b)\text{ are factors} \end{gathered}`

STEP 3: Get the equation

Since we know the roots, we write them in a factor form as seen above to have:

`\begin{gathered} (x-(2+i))---factor1 \\ (x-(2-i))---factor2 \end{gathered}`

We multiply the two factors to get the quadratic equation

`\begin{gathered} \left(x-\left(2+i\right)\right)\left(x-\left(2-i\right)\right) \\ \mathrm{Apply\:FOIL\:method}:\quad \left(a+b\right)\left(c+d\right)=ac+ad+bc+bd \\ \left(x-\left(2+i\right)\right)\left(x-\left(2-i\right)\right)=xx+x\left(-\left(2-i\right)\right)-\left(2+i\right)x-\left(2+i\right)\left(-\left(2-i\right)\right) \\ =xx+x\left(-\left(2-i\right)\right)-\left(2+i\right)x-\left(2+i\right)\left(-\left(2-i\right)\right) \\ By\text{ }simplification: \\ 5+x^2-4x \\ By\text{ rewriting, we have:} \\ x^2-4x+5 \end{gathered}`

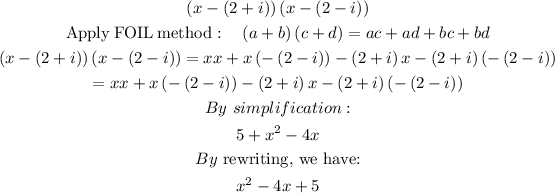
Hence, the answer is:

`x^(2)-4x+5`