Answer:
Answer is C
Explanation:
Area of an equilateral triangle can be found by the following formula,
A=
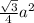
Where "a" is the length of one side of the triangle.
Now we can substitute the value given to the equation above and find the area of the given equilateral triangle.
A=
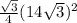
=
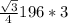
=
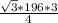
=
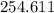
A=
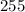 square feet.
square feet.
Answer is C