Question

The length of a rectangle is 4 inches more than its width. The area of the rectangle is equal to 4 inches less than 5 times the perimeter. Find the length and width of the rectangle. SOME HELP WITH THIS PLEASE

Answer 1

Let the width be w, then the length is w+4 (in).

The area of the rectangle is
`A=w(w+4)= w^(2)+4w`.

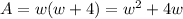
The perimeter of the rectangle is:

P = 2(Width + Length)=2(w+w+4)=2(2w+4)=4w+8

"The area of the rectangle is equal to 4 inches less than 5 times the perimeter." means that:

A = 5P - 4


`w^(2)+4w=5(4w+8)-4`


`w^(2)+4w=20w+40-4`


`w^(2)-16w-36=0`

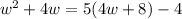
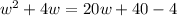
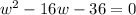
add and subtract 64 to complete the square:


`w^(2)-16w+64-64-36=0`


`(w^(2)-16w+64)=100`


`(w-8)^(2)=10^(2)`

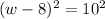
thus w= 18 or w=-2, but the width clearly cannot be negative.

w=18, the length is 18+4 = 22 (in)

Answer:

w=18 in, l=22 in