Question

#13The area of a rectangle is 78 square inches. If the length of the rectangleis one more than twice the width, find the dimensions of the rectangle.

Answer 1

Given

The area of rectangle is given 78 sq.inches.

The length is one more than the twice the width.

Step-by-step explanation

To determine the dimensions of the rectangle.

Let the length be l and width be w.

Then the area of rectangle is

`A=l* w`

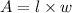
From the statement given, the equation formed is

`l=2w+1`

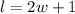
Substitute the length relation in the area of rectangle

`\begin{gathered} A=(2w+1)\cdot w \\ A=2w^2+w \\ 78=2w^2+w \\ 2w^2+w-78=0 \end{gathered}`

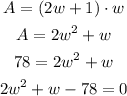
Solve the quadratic equation and find width w.

`\begin{gathered} (w-6)(w+(13)/(2))=0 \\ w=6,(-13)/(2) \end{gathered}`

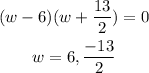
As width cannot be negative then width is 6 in.

And length is determined by

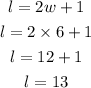
`\begin{gathered} l=2w+1 \\ l=2*6+1 \\ l=12+1 \\ l=13 \end{gathered}`Answer

Hence the length is 13 in and width is 6 in.