Question

Calculate the speed of a proton that is accelerated from rest through an electric potential difference of 163 v. (b) calculate the speed of an electron that is accelerated through the same potential difference.

Answer 1

You are given the electric potential difference of a proton at 163V. You are asked to find the speed of a proton from rest and when moving but the same potential difference. Use the energy of photon equation.

from rest with potential difference of 163 V
E = 1/2 mv² = eΔV
v = √
`\sqrt{ (2e(deltaV))/( m_(p) ) } = \sqrt{ (2 * 1.6 x 10^(-19) *163 )/(1.67 x 10^(-27) ) }`
v = 176,730 m/s or 176.73 km/s

electron that is accelerated through the same potential difference
E = 1/2 mv² = eΔV
v = √
`\sqrt{ (2e(deltaV))/( m_(e) ) } = \sqrt{ (2 * 1.6 x 10^(-19) *163 )/(9.11 x 10^(-31) ) }`
v = 7566753 m/s = 7,566.8 km/s

Answer 2

We could use the energy conservation:

Kinetic energy = Potential energy
=>
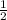
`(1)/(2)` M
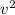
`v^(2)` = eV
where, M = mass of proton
v = speed
e = charge
V = potential difference through which it is accelerated

So, finding speed using the above equation:

v =
`\sqrt{(2eV)/(M)}`

Putting values,
v =
`\sqrt{(2 * 1.6 * 10^(-19) * 163)/(1.67 * 10^(-27))}`
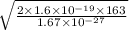
v = 177.15 Km/s

Now, let m = mass of electron

So, Above we got the formula for the speed of proton accelerated through V.

For electron, just replace the mass M (proton) with m (electron) and that's it. Because V is same for both.

So, Speed of electron, v =
`\sqrt{(2eV)/(m)}`

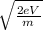
Putting values.

v =
`\sqrt{(2 * 1.6 * 10^(-19) * 163)/(9.1 * 10^(-31))}`

On solving
v = 7.56 x
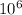
`10^(6)` m/s or 7560 km/s