Question

A rectangle has a length of the cube root of 81 inches and a width of 3 to the 2 over 3 power inches. Find the area of the rectangle.

3 to the 2 over 3 power inches squared
3 to the 8 over 3 power inches squared
9 inches squared
9 to the 2 over 3 power inches squared

Answer 1

`A= \sqrt[3]{81}*3^{ (2)/(3) }= \sqrt[3]{3^4}*3^{ (2)/(3) } = 3^{ (4)/(3) }*3^{ (2)/(3) }=3^{ (4)/(3) + (2)/(3) }=3^2=9 \`

9 inches squared

Answer 2

9 square inches.

Explanation:

We have been given that a rectangle has a length of the
`\sqrt[3]{81}` inches and a width of
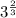`3^{(2)/(3)}` power inches. We are asked to find the area of given rectangle.

We know that area of rectangle in length times width of rectangle.

`\text{Area of rectangle}=\sqrt[3]{81}* 3^{(2)/(3)}`

We can write 81 as
`3^4` as:

`\text{Area of rectangle}=\sqrt[3]{3^4}* 3^{(2)/(3)}`

Using exponent rule
`\sqrt[n]{a^m}=a^{(m)/(n)}`, we can write
`\sqrt[3]{3^4}=3^{(4)/(3)}`.

`\text{Area of rectangle}=3^{(4)/(3)}* 3^{(2)/(3)}`

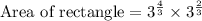
Using exponent rule
`a^b\cdot a^c=a^(b+c)`, we will get:

`\text{Area of rectangle}=3^{(4)/(3)+(2)/(3)}`

`\text{Area of rectangle}=3^{(4+2)/(3)}`

`\text{Area of rectangle}=3^{(6)/(3)}`

`\text{Area of rectangle}=3^(2)`

`\text{Area of rectangle}=9`

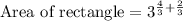
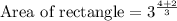
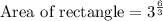
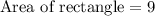
Therefore, the area of given rectangle is 9 square inches.