Question

1280 - 640 + 320 -...+ 5find the sum of the series

Answer 1

855

Explanation:

Given the series:

`1280-640+320-\cdots+5`

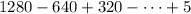
From observation, the series is geometric.

`\begin{gathered} -(640)/(1280)=-(1)/(2) \\ (320)/(-640)=-(1)/(2) \end{gathered}`

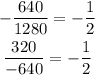
• The first term of the series, a = 1280

,

• The common ratio, r =-1/2

Since the series is finite, we find the number of terms in the series using the formula for the nth term of a geometric series:

`\begin{gathered} U_n=ar^(n-1) \\ 5=1280\left(-(1)/(2)\right)^(n-1) \\ (5)/(1280)=\left(-(1)/(2)\right)^(n-1) \\ (1)/(256)=\left(-(1)/(2)\right)^(n-1) \\ (1)/(2^8)=\left(-(1)/(2)\right)^(n-1) \\ 2^(-8)=(-2)^(-1(n-1)) \\ \text{ Assume n is odd} \\ 2^(-8)=(2)^(-(n-1)) \\ -8=-n+1 \\ n=1+8 \\ n=9 \end{gathered}`

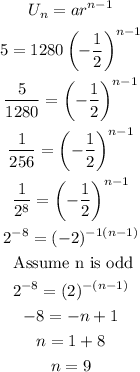
This means that there are 9 terms in the series.

For a geometric series with a common ratio of less than 1, the sum is calculated using the formula:

`S_n=(a(1-r^n))/(1-r)`

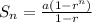
Substitute the values: a=1280, r=-1/2 and n=9

`\begin{gathered} S_9=(1280\left(1-\left(-(1)/(2)\right)^9\right))/(1-\left(-(1)/(2)\right)) \\ =(1280\left(1-\left(-(1)/(512)\right)\right))/(1+(1)/(2)) \\ =(1280\left(1+(1)/(512)\right))/(1+(1)/(2)) \\ =855 \end{gathered}`

The sum of the series is 855.