Question

I REALLY NEED HELP ON THESE EQUATIONS THE LAST PIC AND THIS ONE PLEASEEE IM IN DANGER OF FAILING

Answer 1

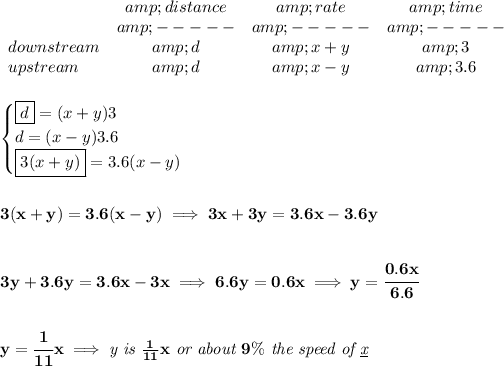
d = rt, distance = rate * time

so, we know the boat is going at a rate of "x", and the current has a rate of "y".

ok... from A to B, the boat is going downstream, the speed of the boat is not really "x", is " x + y ", because, is going with the stream and thus the current is adding "y" to its speed.

now, from B to A, the boat is going upstream, against the current, is not really going "x" fast either, is going " x - y ", because the current is eroding/subtracting from it's speed.

ok... since, since the towns A and B didn't really move, unless they're floating towns, but they're not, so, the distance from A to B is the same distance from B to A, say they're at a distance "d".


`\bf \begin{array}{lccclll} &distance&rate&time\\ &-----&-----&-----\\ downstream&d&x+y&3\\ upstream&d&x-y&3.6 \end{array} \\\\\\ \begin{cases} \boxed{d}=(x+y)3\\ d=(x-y)3.6\\ \boxed{3(x+y)}=3.6(x-y) \end{cases} \\\\\\ 3(x+y)=3.6(x-y)\implies 3x+3y=3.6x-3.6y \\\\\\ 3y+3.6y=3.6x-3x\implies 6.6y=0.6x\implies y=\cfrac{0.6x}{6.6} \\\\\\ y=\cfrac{1}{11}x\implies \textit{y is }(1)/(11)x\textit{ or about }9\%\textit{ the speed of \underline{x}}`