Question

A family has 8 girls and 4 boys. A total of 2 children must be chosen to speak on the behalf of the family at a local benefit. What is the probability that 2 girls and no boys are chosen?

A. 7/55
B. 14/33
C. 12/33
D. 1/6

Answer 1

P(GG)=(8/12)(7/11)

P(GG)=56/132

P(GG)=14/33

Answer 2

Answer: The correct option is (B)
`(14)/(33).`

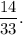
Step-by-step explanation: Given that a family has 8 girls and 4 boys. A total of 2 children must be chosen to speak on the behalf of the family at a local benefit.

We are to find the probability that 2 girls and no boys are chosen.

Total number of children in the family = 8 + 4 =12.

Let S denote the sample space of choosing 2 children from the family of 12 children and A denote the event of choosing 2 girls and no boys.

Then, according to the given information, we have

`n(S)=^(12)C_2=(12!)/(2!(12-2)!)=(12*11*10!)/(2*1*10!)=66,\\\\\\n(A)=^8C_2*^4C_0=(8!)/(2!(8-2)!)*1=(8*7*6!)/(2*1*6!)=28.`

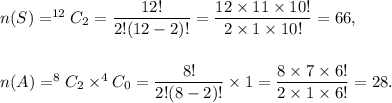
Therefore, the probability of event A is given by

`P(A)=(n(A))/(n(S))=(28)/(66)=(14)/(33).`

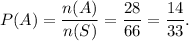
Thus, the required probability is
`(14)/(33).`

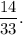
Option (B) is CORRECT.