Answer: Equation of line in point slope form,
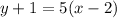
And, Equation of line in standard form,
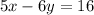
Explanation:
Since, If a line passes through two points
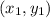 and
and
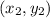 ,
,
Then the equation of line,

Here
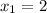 ,
,
 ,
,
 and
and

Thus, the equation of the given line,

⇒
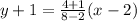
⇒
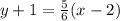 -----(1)
-----(1)
⇒
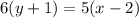
⇒ 6 y + 6 = 5 x - 10
⇒ 6 = 5x - 6y - 10 ( By subtracting by on both sides )
⇒ 6 + 10 = 5x - 6y ( By adding 10 on both sides )
⇒ 16 = 5x - 6y
⇒ 5 x - 6 y = 16 ------(2)
Since, in slope for of a line is,
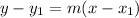
Thus, equation (1) shows the in slope form of the line.
And, standard form of the line is ax + by = c where a, b and c are the integers.
Thus, equation (2) shows the standard form of the given line.