Question

What are the x-intercepts of the graph of the function f(x) = x2 + 4x – 12?

(–6, 0), (2,0)
(–2, –16), (0, –12)
(–6, 0), (–2, –16), (2, 0)
(0, –12), (–6, 0), (2, 0)

Answer 1

A

Explanation:

Answer 2

x-intercepts are (-6,0 and (2,0)

First option is correct.

Explanation:

The given equation is
`y=x^2+4x-12`

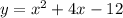
For x-intercept, y=f(x)=0. Thus, we have

`0=x^2+4x-12`

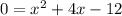
We can split the middle term as 4x = 6x-2x

Thus, the equation becomes

`x^2+6x-2x-12=0`

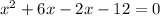
Now, we make group

`(x^2+6x)+(-2x-12)=0`

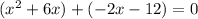
Take GCF from each of the group

`x(x+6)-2(x+6)=0`

Factored out the common term

`(x+6)(x-2)=0`

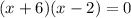
Apply the zero product property,

`x+6=0,x-2=0`

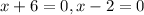
Solve for x

`x-6,x=2`

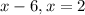
Thus, x-intercepts are (-6,0 and (2,0)

First option is correct.