Answer:
six divided by the square root of thirteen
Explanation:
hey there,
< sin θ =
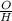
So that means O = 2 and H = 3. In order to find cot θ, first let's find tan θ.
tan θ =
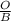
We only know what O is equal to, not B. So let's draw out a triangle.
7
◢ 6
B
As you can see (sorry for the poor triangle), this is a right triangle. In order to find an unknown part, use
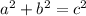 !
!

B = ±√13
Obviously, a side of a triangle can't be negative, so it stays positive. Now we can find tangent!
tanθ =
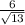
But, we're not done here. We're trying to find cotθ.
cotθ =
 θ
θ
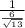 =
=
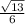
That's your final answer! >
Hope this helped! Feel free to ask anything else.