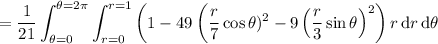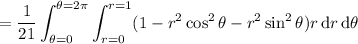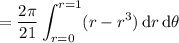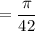The line integral is given by
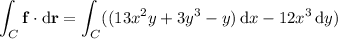
By Green's theorem, the line integral along
is equivalent to the double integral over
(the region bounded by
)

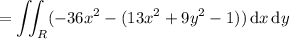
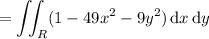
Now consider the function

. We can think of the double integral above as a volume integral; namely, it's the volume of the region below

and above the region
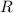
in the

-

plane (i.e.

). This volume will be maximized if
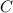
is taken to be the intersection of

with the plane, which means
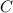
is the ellipse
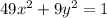
.
For the double integral, we can convert to an augmented system of polar coordinates using

where
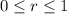
and
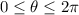
. We have the Jacobian determinant
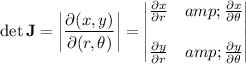
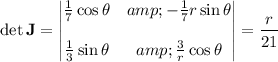
So the double integral, upon converting to our polar coordinates, is equivalent to