The expression is:
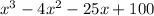
We know that one of the zeros of this expression is x=-5. We have to find the others.
Then, we can write this expression as:
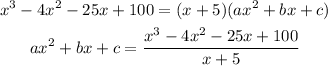
We have to perform the polynomial division:

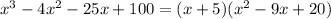
Now, we can calculate the zeros of the quadratic polynomial as:
![\begin{gathered} x=\frac{-(-9)\pm\sqrt[]{(-9)^2-4\cdot1\cdot(20)}}{2\cdot1} \\ x=\frac{9\pm\sqrt[]{81-80}}{2} \\ x=\frac{9\pm\sqrt[]{1}}{2} \\ x_1=(9-1)/(2)=(8)/(2)=4 \\ x_2=(9+1)/(2)=(10)/(2)=5 \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/nxbuauwhhqribq7xjncm.png)
Answer: the other two zeros are x=4 and x=5