Question

Write an equation for the translation of the function. y = cos x; translated 6 units up

Answer 1

it's just y= cos(x)+6

Answer 2

`y=cos(x)+6`.

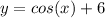Explanation:

We are asked to write an equation for the translation of the function
`y=cos(x)` to 6 units upwards.

Let us recall transformation rules.

`f(x)\rightarrow f(x+a)=\text{Graph shifted to left by a units}`

`f(x)\rightarrow f(x-a)=\text{Graph shifted to right by a units}`

`f(x)\rightarrow f(x)+a=\text{Graph shifted upwards by a units}`

`f(x)\rightarrow f(x)-a=\text{Graph shifted downwards by a units}`

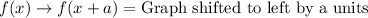
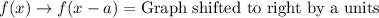
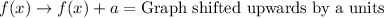
Since we need to shift our given function 6 units upwards, so we will add 6 to our given function outside the parenthesis as:

`y=cos(x)+6`

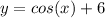
Therefore, our required equation would be
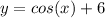`y=cos(x)+6`.