Question

which of the following correctly describes the end behavior of the polynomial function, f(x)=3x^4+2x^2-x

Answer 1

Answer:both ends go down !

Explanation:

Answer 2

`f(x)\rightarrow +\infty\text{ as }x\rightarrow -\infty`

`f(x)\rightarrow +\infty\text{ as }x\rightarrow +\infty`

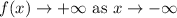
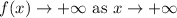
Explanation:

Given polynomial function is,

`f(x)=3x^4+2x^2-x`

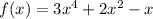
Since, the end behavior of a polynomial is same as the end behavior of leading term,

Here, the leading term =
`3x^4`

`\text{As }x\rightarrow -\infty`

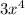
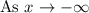
The leading term is positive,

`\text{While, as }x\rightarrow +\infty`

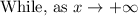
The leading term is positive,

Hence, the end behavior of the given polynomial is,

`f(x)\rightarrow +\infty\text{ as }x\rightarrow -\infty`

`f(x)\rightarrow +\infty\text{ as }x\rightarrow +\infty`

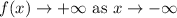
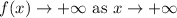
⇒ In the graph of f(x), both ends will go upward.