Question

At 600.0 K the rate constant is 6.1× 10–8 s–1. What is the value of the rate constant at 800.0 K?

Answer 1

The rate constant from the Arrhenius equation is

`k=Ae^{-E_(a)/(RT)}}`
where
A = frequency factor
Ea = activation energy. It is assumed to be 50 kJ/mol, because it s not given.
R = gas constant = 8.31 J/(K-mol)
T = temperature, K

Evaluate Ea/R = 50000/8.31 = 6.0168 x 10³ K.
Therefore

`k=Ae^{-6.0168*10^(3)/T}`

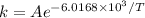
When T₁ = 600 K, k₁ = 6.1 x 10⁻⁸ s⁻¹
Therefore when T₂ = 800 K, the rate constant is

`(k_(2))/(6.1*10^(-8))= (e^(-6016.8/800))/(e^(-6016.8/600)) \\ =e^(-6016.8(1/800-1/600))\\ =e^(2.507)\\ =12.268`
k₂ = 7.484 x 10⁻⁷ s⁻¹
The rate has increased by a factor of about 12.

Answer: The rate constant is approximately 7.5 x 10⁻⁷ s⁻¹