Question

What are the sine, cosine, and tangent of Θ = 7 pi over 4 radians?

sin Θ = negative square root 2 over 2; cos Θ = negative square root 2 over 2; tan Θ = 1

sin Θ = negative square root 2 over 2; cos Θ = square root 2 over 2; tan Θ = 1

sin Θ = square root 2 over 2; cos Θ = negative square root 2 over 2 tan Θ = −1

sin Θ = negative square root 2 over 2; cos Θ = square root 2 over 2; tan Θ = −1

Answer 1

d

Explanation:

test

Answer 2

Option D

Explanation:

We are given an angle as

`\theta =(7\pi )/(4)`

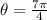
This angle lies between 3pi/2 and 2pi

i.e. in IV quadrant

In Iv quadrant only cos and secant are positive

sine and tangent would be negative

The principal value is pi/4 and for this tan =1

Hence we have

sin Θ = negative square root 2 over 2; cos Θ = square root 2 over 2; tan Θ = −1

Option D is right