Answer: 10 square units.
Explanation:
The area of triangle with vertices
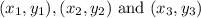 is given by :-
is given by :-
![A=(1)/(2)[x_1(y_2-y_3)+x_2(y_3-y_1)+x_3(y_1-y_2)]](https://img.qammunity.org/2018/formulas/mathematics/college/j5ulv18vymn4b7w4ucb7bk7f9a3j9hnq2g.png)
Given : The vertices of triangle : (2,0), (6,0), (8,5)
Then , the area of the triangle will be :_
![A=(1)/(2)[(2)((0)-(5))+(6)((5)-(0))+(8)((0)-(0))\\\\\Rightarrow A=(1)/(2)[20]\\\\\Rightarrow A=10\text{ square units}](https://img.qammunity.org/2018/formulas/mathematics/college/a3e3egr703smlehrn6o670wigygaj7c3fr.png)
Hence, the number of square units in the area of the triangle whose vertices are points (2,0), (6,0), (8,5) = 10