Question

Simplify open parentheses x to the 2 fifths power close parentheses to the 5 sixths power.

Answer 1

`\left(x^{ (2)/(5) } \right)^{ (5)/(6) }=x^{ (2)/(5)* (5)/(6) }=x^{ (1)/(3) }= \sqrt[3]{x}`

Answer 2

`x^{(1)/(3)}`.

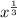Explanation:

We have been given an expression
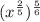`(x^{(2)/(5)})^{(5)/(6)}`. We are asked to simplify our given expression.

Using Power Rule of exponents
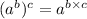`(a^b)^c=a^(b* c)`, we can rewrite our expression as:

`x^{(2)/(5)*(5)/(6)}`

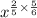
Upon simplifying our expression, we will get:

`x^{(10)/(30)}`

`x^{(1)/(3)}`

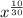
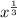
Therefore, the simplified form of our given expression would be
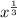`x^{(1)/(3)}`.