we can write a equation for each statement
The receipts were $3331.50
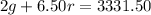
where g is the number of general ticket and r the number of reserverd ticket
930 paid admissions
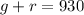
we have two equations, we can solve a variable for the second equation an replace on the firts, i will solve g
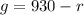
now replace on the first equation to solve r
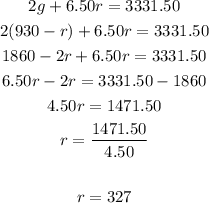
the number of reserved tickets is 327
now replace r=327 on the second equation to find g

the number of general tickets is 603