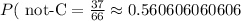Question

Giving a test to a group of students, the grades and gender are summarized belowABCTotalMale371828Female8191138Total11262966If one student is chosen at random,Find the probability that the student did NOT get an "C"

Answer 1

We have the following two-way table (or contingency table) as follows:

And we need to determine the probability that the student did NOT get a "C".

To find it, we can proceed as follows:

1. We have to find the probability of getting a "C" according to the table. We can see that the total of students that get a "C" was 29 (for this event).

2. We can also see that the total of students is 66. Then the probability of getting a "C" is:

`P(C)=(29)/(66)`

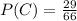
3. Since we need to find the probability of NOT getting a "C", then we have to find the probability of the complement to that probability, that is:

`\begin{gathered} P(\text{ not-C\rparen}=1-P(C) \\ \\ P(\text{ not-C\rparen}=1-(29)/(66)=(66)/(66)-(29)/(66)=(37)/(66)\approx0.560606060606 \\ \\ \end{gathered}`

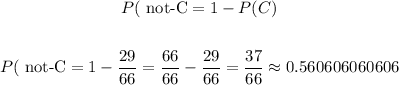
Therefore, in summary, the probability that the student did NOT get a "C" is:

`P(\text{ not-C\rparen}=(37)/(66)\approx0.560606060606`