Question

What is the simplified form of the quantity of x plus 7, all over the quantity of 6 − the quantity of x plus 5, all over the quantity of x plus 3?

Answer 1

assuming the question looks like this:

(x+7)/6 - (x+5)/(x+3)

multiply by (x+3):

(x+7)(x+3)/6 - (x+5)

multiply by 6:

(x+7)(x+3) - 6(x+5)

expand:

x^2 + 10x + 21 - 6x - 30

collect like terms:

x^2 + 4x - 9

hope that helps :)

Answer 2

The quantity of x squared plus 4x minus 9, all over 6 times the quantity of x plus 3

Explanation:

Given expression,

The quantity of x plus 7, all over the quantity of 6 − the quantity of x plus 5, all over the quantity of x plus 3,

`\implies (x+7)/(6)-(x+5)/(x+3)`

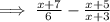
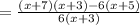`=((x+7)(x+3)-6(x+5))/(6(x+3))` ( Subtraction of fractions ),

`=(x(x+3)+7(x+3)-6x-30)/(6(x+3))` ( Distributive property )

`=(x^2+3x+7x+21-6x-30)/(6(x+3))`

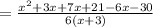
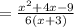`=(x^2+4x-9)/(6(x+3))` ( Combine like terms ),

= The quantity of x squared plus 4x minus 9, all over 6 times the quantity of x plus 3