By the binomial theorem, which says
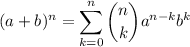
where
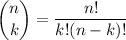
, the seventh term of the expansion is given when

. Take
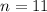
,

, and
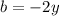
, and we get

Note: it's possible that you may know the binomial theorem in the reverse direction, which says
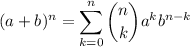
in which case the answer would be
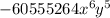
.