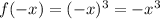Question

Which rule should be applied to reflect f(x) = x3 over the y-axis?

A. Substitute -x for x and simplify f(-x)
B. Multiply f(x) by -1.
C. Switch the variables x and y in the equation
D. Multiply f(y) by -1.

Answer 1

Explanation:

The answer is C. Switch the variables x and y in the equation.

The person above me said It was A and it was a verified answer but I put it in and it said it was wrong and that is in fact C not A.

Answer 2

A. Substitute -x for x and simplify f(-x)

Explanation:

While reflecting a point with coordinates as (x, y), over y axis, the rule followed is

`(x,y)\rightarrow (-x,y)`

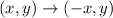
Here, the sign of only x coordinate is changed while y coordinate remains unchanged.

The given function is,

`f(x)=x^3`

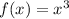
So, reflecting it over y axis, we have to replace x with -x, i.e
`(x,f(x))\rightarrow (-x,f(x))`

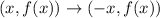
Hence the new reflected function is,

`f(-x)=(-x)^3=-x^3`