Answer:
We have:
Explanation:
We are given angle F as:
m∠GF=87°
Now, g=28 and h=15 we are asked to find f.
Using the law of cosines we have:
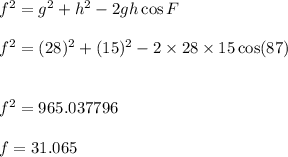
which is approximately equal to 31 units
Hence, f=31 units
Also,
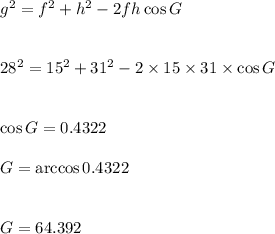
Hence, to the nearest degree we get:
m∠G=64°
Also, we know that the sum of all the angles of a triangle is 180°
Hence,
m∠F+m∠G+m∠H=180°
i.e.
87°+64+m∠H=180°
m∠H=29°