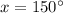Answer:
Option A is correct.
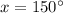
Explanation:
It is given that
 is parallel to
is parallel to
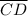
To find the value of x:
labelled the diagram as shown in the attachment:

 {Vertically opposite angle}
{Vertically opposite angle}
Corresponding angles theorem states: If two parallel lines are cut by a transversal, then the pairs of corresponding add up to 180 degree.
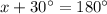
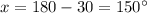
therefore, the value of