Question

An initial population of 7 chipmunks in Mary's yard increases by 6% each year. If the function f(x) = abx models this situation, what is the population of the chipmunks in 5 years? (to the nearest whole number)

A) 9 chipmunks
B) 11 chipmunks
C) 15 chipmunks
D) 20 chipmunks

Answer 1

The answer for this qn is A

Answer 2

A. 9 chipmunks

Explanation:

We are given that,

The initial population of chipmunks = 7

The rate of increase = 6% = 0.06

Since, the exponential growth function is modeled by,
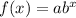`f(x)=ab^x`, where
`b=1+r`, 'r' = rate of increase and 'x' is the number of years.

So, we have, on comparing,

`a=7\\b=1+0.06=1.06`

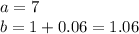
Then, the function modeling the given situation is,
`f(x)=7(1.06)^x`.

So, the population after 5 years is given by,

`f(5)=7(1.06)^5`

i.e.
`f(5)=7* 1.34`

i.e. f(5) ≈ 9.

Thus, there are 9 chipmunks after 5 years.

Hence, option A is correct.