Let the weight (in kg) of a large box be 'x' and the weight of a small box be 'y'.
Given that the weight of 2 large boxes and 3 small boxes is 78 kg,
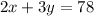
Also given that the weight of 6 large boxes and 5 small boxes is 180 kg,
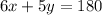
Here we will apply the Elimination Method to solve both the equations.
Consider the three times the first equation being subtracted from the second equation,
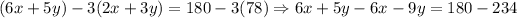
Simplify the terms,
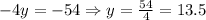
Substitute this value in the first equation,
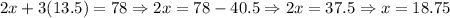
Thus, the larger box weighs 18.75 kg while the small box weighs 13.5 kg