Question

Find the solution of sqrt x+3+4=6, and determine if it is an extraneous solution.

Answer 1

I'm assuming you mean
`√(x+3)+4=6`
First, isolate the radical so you have
`√(x+3) =6-4`

`√(x+3) =2`
Then, square both sides
`x+3=2^(2)`

`x+3=4`

`x=1`

Answer 2

x=1 and is not extraneous.

Explanation:

We have been given the equation:

`√(x+3)+4=6`

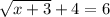
collect terms without square root so, we will shift 4 on the right hand side of the equation.

Hence, equation becomes:

`√(x+3)=6-4`

`√(x+3)=2`

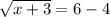
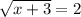
Now, squaring both sides so, as to remove square root we get:

`{x+3}=4`

`x=1`

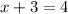
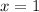
At x=1 is not extraneous because extraneous is the point where we get zero at that point in a function.