Question

What is the area of this triangle?

A.) A=1/2(y3-y2)(x3-x1)
B.) A=1/2(y3-y1)(x3-x1)
C.) A=1/2(y3-y1)(x2-x1)
D.) A=1/2(y2-y1)(x3-x1)
E.) A=1/2(y2-y1)(x2-x1)

Answer 1

The answer is D.) A=1/2(y2-y1)(x3-x1).
Step-by-step explanation:
Area of a triangle equals side×height.
In this case height is x3-x1, and side y2-y1.

Answer 2

Option D is correct

Step-by-step explanation:

`\text{Given a triangle whose vertices are }(x_1, y_1), (x_1, y_2) , (x_3, y_3)`

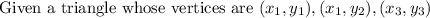
we have to find the area of triangle.

As we know area of triangle can be calculated by the formula

`(1)/(2)* base* height`

Base of triangle is the line joining the coordinates
`(x_1, y_1), (x_1, y_2)` whose length is =
`(y_2-y_1)`

Now height of triangle is the perpendicular line from the vertex
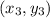`(x_3, y_3)` whose length is
`(x_3-x_1)`

Hence, area of triangle is

`(1)/(2)* (y_2-y_1)* (x_3-x_1)`

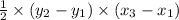
Option D is correct