Question

A very old tree limb contains an amount of carbon-14 that is approximately 1/8 of the current atmospheric 14C levels.. Calculate your answers to the following and be sure to show all steps of your calculations.

• Approximately how long ago was the tree cut down?
• If there were 1,500,000 carbon-14 atoms to begin with, how many atoms would remain

Answer 1

A. The radioactive decay equation is N = N0
`e^(-ln(2)*t/T )`

where T is the half-life (5730 years), N0 is the number of atoms at time t = 0 and N is the number at time t.

Rewriting this as:

(N/N0) =
`e^(-ln(2)*t/T )`

Since N = (1/8) N0 and substituting known values:

1/8 =
`e^(-ln(2)*t/5730)`

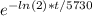
Taking ln of both sides:

ln(1/8)= -ln(2)*t/5730

t = - 5730 * ln(1/8) / ln (2)

t = 17,190 years

The tree was cut down 17,190 years ago.

B. N0 = 1,500,000 carbon-14 atoms

Since N = (1/8) N0

N = 187,500 carbon atoms left