Answer:
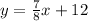 and
and

Explanation:
The product of the slopes of the perpendicular lines is -1
Option 1)
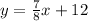 and
and

General equation of line :

Comparing with general equation
Slope of line 1 =
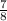
Slope of line 2=

Now product of slopes =
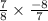
=
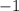
Since The product of the slopes of the perpendicular lines is -1
So,
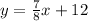 and
and
 represent perpendicular lines
represent perpendicular lines
Option 2)
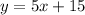 and
and
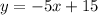
General equation of line :

Comparing with general equation
Slope of line 1 = 5
Slope of line 2= -5
Now product of slopes =
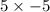
=
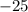
Since The product of the slopes of the perpendicular lines is not -1
So,
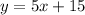 and
and
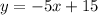 does not represents the perpendicular lines.
does not represents the perpendicular lines.
Option 3)
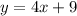 and
and
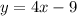
General equation of line :

Comparing with general equation
Slope of line 1 = 4
Slope of line 2= 4
Now product of slopes =

=
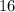
Since The product of the slopes of the perpendicular lines is not -1
So,
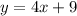 and
and
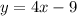 does not represents the perpendicular lines.
does not represents the perpendicular lines.
Option 4)
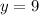 and
and
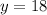
General equation of line :

Comparing with general equation
Slope of line 1 = 0
Slope of line 2=0
Now product of slopes =
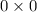
=
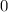
Since The product of the slopes of the perpendicular lines is not -1
So,
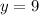 and
and
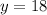 does not represents the perpendicular lines.
does not represents the perpendicular lines.