INFORMATION:
We know that:
- 34.37 g of tin(IV) phosphate is added to 34.05 g of sodium carbonate
- 24.24 g of tin(IV) carbonate are made
And we must find the %yield
STEP BY STEP EXPLANATION:
Balanced equation:
Sn3(PO4)4 + 6Na2CO3 → 3Sn(CO3)2 + 4Na3PO4
1. We must find the amount of tin carbonate produced by tin phosphate
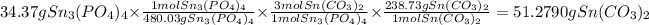
2. We must find the amount of tin carbonate produced by sodium carbonate
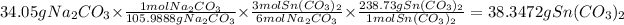
3. We must find the theoretical amount of tin carbonate produced
Since sodium carbonate is the limiting reactant, then the theoretical amount of tin carbonate produced would be
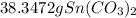
4. Finally, the %yield would be
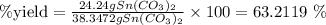
ANSWER:
1.
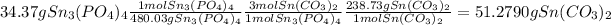
2.
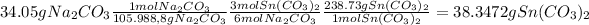
3.
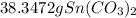
4.
![\operatorname{\%}\text{y}\imaginaryI\text{eld}=(24.24gSn(CO_(3))_(2))/(38.347,2gSn(CO_(3))_(2))*100=63.2119\operatorname{\%}]()