In this problem, the given values are:
x = average = 30.4
s = standard deviation = 7.1
n = number of samples = 25
Degrees of freedom = n -1 =24
Using the t-distribution table for a normal curve at 90% CI, we get t-crit:
t-crit = 1.711
Now the Margin of Error (E) is calculated as:
E = t-crit*(s/
 )
)
By substituting the known values into the equation:
E = 1.711 * (7.1/
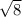 )
)
E = +- 4.30
Therefore, the margin of error is 4.36. Hence, the commute time is between 26.1 minutes and 34.7 minutes.